Rankine vortex

The Rankine vortex model is an attempt to describe the velocity profile through vortices in real, viscous, fluids. It is named after its creator, William John Macquorn Rankine.

A swirling flow in a viscous fluid is characterized by a forced vortex in the central core, surrounded by a free vortex. The Rankine vortex best describes this phenomenon. (The velocity profile through an ideal vortex in an inviscid fluid consists entirely of the free vortex with an infinite velocity at its centre. There is no low velocity core.)

The tangential velocity^[1] of a Rankine vortex with circulation 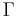$\Gamma$ and radius $R$ is

$u_\theta(r) = \begin{cases} \Gamma r/(2 \pi R^2) & r \le R, \\ \Gamma/(2 \pi r) & r > R. \end{cases}$

The remainder of the velocity components are identically zero, so that the total velocity field is $\mathbf{u} = u_\theta\ \mathbf{e_\theta}$ .

External links

Streamlines vs. Trajectories in a Translating Rankine Vortex An example of a Rankine vortex imposed on a constant velocity field, with animation.

Notes

^ D. J. Acheson (1990). Elementary Fluid Dynamics. Oxford University Press. ISBN 0198596790.

Rankine vortex

See also

External links

Notes